Research Areas
Present, future, and past activities: We carry out research in several areas of geometry, linking applied and pure science.
|
|
 We aim to understand (geometric) methods, and their interrelations, to generate and design (geometric) shapes, e.g., shape generation by means of representation formulae, by transformations, kinematic generation methods, etc.
We aim to understand (geometric) methods, and their interrelations, to generate and design (geometric) shapes, e.g., shape generation by means of representation formulae, by transformations, kinematic generation methods, etc.
|
 This project is devoted to evaluating the closeness of Stewart-Gough platforms to singularities.
This project is devoted to evaluating the closeness of Stewart-Gough platforms to singularities.
|
|
 The topic of our research are connections among spreads and parallelisms of projective spaces with areas like the geometry of field extensions, topological geometry, kinematic spaces, translation planes or flocks of quadrics.
The topic of our research are connections among spreads and parallelisms of projective spaces with areas like the geometry of field extensions, topological geometry, kinematic spaces, translation planes or flocks of quadrics.
|
 We will investigate relations between non-rigidity and symmetry breaking, in particular, whether deformability in more than one way invariably leads to symmetry breaking.
We will investigate relations between non-rigidity and symmetry breaking, in particular, whether deformability in more than one way invariably leads to symmetry breaking.
|
|
 The main aim of the project is the systematic determination, investigation and classification of Steward-Gough platforms with self-motions.
The main aim of the project is the systematic determination, investigation and classification of Steward-Gough platforms with self-motions.
|
 We aim to understand how transformations of particular surfaces behave (or fail to behave) at singularities, and to study how those transformations create (or annihilate) singularities.
We aim to understand how transformations of particular surfaces behave (or fail to behave) at singularities, and to study how those transformations create (or annihilate) singularities.
|
|
 The aim of the project is to investigate various aspects of the generalised chain geometry arising from the projective line over a ring which contains a (possibly skew) field.
The aim of the project is to investigate various aspects of the generalised chain geometry arising from the projective line over a ring which contains a (possibly skew) field.
|
 We investigate polyhedra and frameworks which are flexible or infinitesimally flexible, and problems related to the bellows conjecture.
We investigate polyhedra and frameworks which are flexible or infinitesimally flexible, and problems related to the bellows conjecture.
|
|
 We study differential geometric invariants of ruled surfaces, line congruences, and complexes of lines.
We study differential geometric invariants of ruled surfaces, line congruences, and complexes of lines.
|
 This is a method to study 3D geometry through 2D images. It provides insight into structure and metrical properties of spatial objects, processes and principles. We are interested in new educational tools and methodologies.
This is a method to study 3D geometry through 2D images. It provides insight into structure and metrical properties of spatial objects, processes and principles. We are interested in new educational tools and methodologies.
|
|
The aim of the project is to study projective and affine matrix spaces and related questions in the research field of linear preserver problems.
|
We exhibit the geometry and embeddings of the Grassmann space G(n,d) formed by the d-dimensional subspaces of an n-dimensional projective space over an arbitrary (skew) field F and related topics, like products of Grassmann spaces, Schubert spaces, or flag spaces.
|
|
 We investigate finite combinatorial structures, like designs and divisible designs.
We investigate finite combinatorial structures, like designs and divisible designs.
|
 Our aim is to characterise transformation groups via the invariance of a single geometric notion.
Our aim is to characterise transformation groups via the invariance of a single geometric notion.
|
|
 In affine differential geometry a main point of research is the investigation of special surfaces in three dimensional affine space.
In affine differential geometry a main point of research is the investigation of special surfaces in three dimensional affine space.
|
 For any researcher with a love of geometry, enticing problems remain.
For any researcher with a love of geometry, enticing problems remain.
|
|
 The aim of this project is to discuss the mappings used in Descriptive Geometry and their generalisations from both a synthetic and analytic point of view.
The aim of this project is to discuss the mappings used in Descriptive Geometry and their generalisations from both a synthetic and analytic point of view.
|
 We are interested in flexible and in infinitesimally flexible structures - in theory as well as in applications, like gearing or the design of mechanisms for real world problems.
We are interested in flexible and in infinitesimally flexible structures - in theory as well as in applications, like gearing or the design of mechanisms for real world problems.
|
|
 The aim of this project is to discuss Veronese varieties over a commutative ground field with non zero characteristic.
The aim of this project is to discuss Veronese varieties over a commutative ground field with non zero characteristic.
|
 We investigate reguli, Segre manifolds, normal rational curves, and their generalisations in projective spaces over arbitrary skew fields.
We investigate reguli, Segre manifolds, normal rational curves, and their generalisations in projective spaces over arbitrary skew fields.
|
|
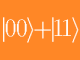 We exhibit, among other topics, finite geometries that describe the commutation relations between generalised Pauli operators and their Kronecker products.
We exhibit, among other topics, finite geometries that describe the commutation relations between generalised Pauli operators and their Kronecker products.
|
Past Activities: Geometric Tolerancing,
Nonlinear Subdivision, Geometric Spline Theory, Discrete Differential Geometry, Developable Surfaces
These topics can be found on the web site of Johannes Wallner, Graz University of Technology (external link).
|





